Answer:
167 pairs
Explanation:
Represent jeans with J and shirts with S
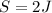
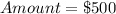 --- Maximum
--- Maximum
Required
Determine the number of pair of jeans he can buy
To represent as an inequality, we have:

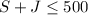
Substitute 2J for S
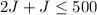
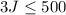
Divide through by 3

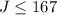
Hence, without exceeding $500, Jess can buy 167 pairs of jeans