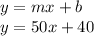Please help me. Look at the picture.

Question

asked Mar 28, 2021 180k views

2 Answers

Jon Vogel · Answer 1 · 2021-04-02T06:31:49+0000

Answer:

Explanation:

To find the equation of the line in slope-intercept form we to find the y-intercept(b) and the slope(m)

For the y-intercept(b) we can see that the line crosses/intersects the y-axis at 40 so the value of b equals 40 which means our y-intercept is 40

now all that is remaining is the value of m which we can find through the formula:

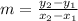
m=(y_2-y_1)/(x_2-x_1)

we take any two coordinates which lie on the line for example

(0 , 40) and (2 , 140) and plug it into our formula

$m=(y_2-y_1)/(x_2-x_1) \\\\m=(140-40)/(2-0)\\\\m=(100)/(2) \\\\m=50$

so the slope of the line is 50 which is m , so the equation of the line in slope-intercept form would be:

$y=mx+b\\y=50x+40$