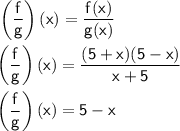Answer:
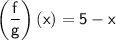
Explanation:
Given functions:
f(x) = 25 - x²
g(x) = x + 5
Difference of Perfect Squares rule: a² - b² = (a + b)×(a - b)
1. Rewrite function f(x) using the rule:
5 × 5 = 25 ⇒ 5²
x × x = x²
f(x) = 5² - x² ⇒ f(x) = (5 + x)×(5 - x)
2. Divide and simplify: