Question

Solve – 6(x – 3) + x + 3 = -9 for x.

Answer 1

In this equation, the value of x is equal to 6.

Explanation:

We are given an algebraic equation in which we need to solve for x.

We can use several properties and algebraic methods to solve this equation.

`\displaystyle -6(x - 3) + x + 3 = -9`

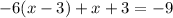
Firstly, we want to use the distributive property to evaluate the first term in the equation: -6(x - 3).

`-6(x - 3) + x + 3 = -9\\\\-6x + 18 + x + 3 = -9`

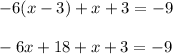
By distributing the -6 through, we get -6x and positive 18. Then, we need to begin combining like terms. Let's start with our constants (or the terms without a variable).

`-6x + 18 + x + 3 = -9\\\\18 + 3 = 21\\\\-6x + x + 21 = -9`

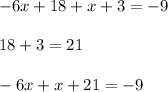
Then, we can combine our terms with variables.

`-6x + x + 21 = -9\\\\-6x + x = -5x\\\\-5x + 21 = -9`

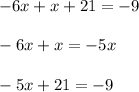
Now, we can use the subtraction property of equality which states that if we subtract a term from one side of an equation, we must also subtract it from the opposite side. Therefore, we want to get x by itself, so we can subtract 21 from both sides using the subtraction property of equality to do this.

`-5x + 21 = -9\\\\-5x + 21 - 21 = -9 -21\\\\-5x = -30`

Finally, we can use the division property of equality and divide both sides of the equation by -5 to isolate x.

`\displaystyle -5x = -30\\\\(-5x)/(-5) = (-30)/(-5)\\\\x = 6`

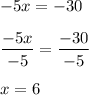
Therefore, by solving the multi-step equation, we solved that x = 6.