Answer:
Approximately
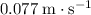 (assuming that external forces on the cannon are negligible.)
(assuming that external forces on the cannon are negligible.)
Step-by-step explanation:
If an object of mass
 is moving at a velocity of
is moving at a velocity of
 , the momentum
, the momentum
 of that object would be
of that object would be
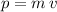 .
.
Momentum of the t-shirt:
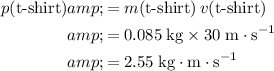 .
.
If there is no external force (gravity, friction, etc.) on this cannon, the total momentum of this system should be conserved. In other words, if
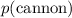 denote the momentum of this cannon:
denote the momentum of this cannon:
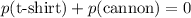 .
.
 .
.
Rewrite
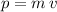 to obtain
to obtain
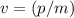 . Since the mass of this cannon is
. Since the mass of this cannon is
 , the velocity of this cannon would be:
, the velocity of this cannon would be:
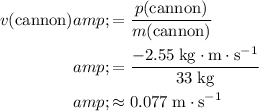 .
.