Question

What is the solution to -3/4x + 2 is less then or equal to -7

Answer 1

its b

Explanation:

x ≥ 12

Answer 2

QUESTION:

What is the solution to -3/4x + 2 is less then or equal to -7

ANSWER:

`\green{{x ≥ 12}}`

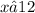
STEP-BY-STEP EXPLANATION:

First, Simplify each term

Combine
`{x}` and
`{(3)/(4)}`

`\green{{-(x × 3)/(4) + 2 ≤ - 7}}`

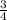
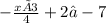
Move 3 to the left of
`{x}`

`\green{{-(3x)/(4) + 2 ≤ - 7}}`

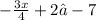
Second, Move all terms not containing
`{x}` to the right side of the inequality

Subtract 2 from the both sides of the inequality

`\green{{-(3x)/(4) ≤ - 7 - 2}}`

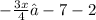
Subtract 2 from - 7

`\green{{-(3x)/(4) ≤ - 9}}`

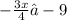
Third, Multiply each term in
`{-(3x)/(4) ≤ - 9 by - 1}`

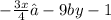
When multiplying or dividing both sides of an inequality by a negative value, flip the direction of the inequality sign

`\green{{-(3x)/(4) × - 1 ≥ ( - 9) × - 1}}`

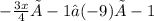
Fourth, Multiply
`{-(3x)/(4) × - 1}`

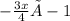
Multiply - 1 by - 1

`\green{{1(3x)/(4) ≥ ( - 9) × - 1}}`

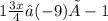
Multiply
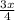`{(3x)/(4)}` by 1

`\green{{(3x)/(4) ≥ ( - 9) × 1}}`

Multiply - 9 by - 1

`\green{{(3x)/(4) ≥ 9}}`

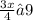
Multiply both sides of the equation by 4

`\green{{3x ≥ 9 × (4)}}`

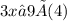
Multiply 9 by 4

`\green{{3x ≥ 36}}`

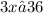
Fifth, Divide each term by 3 and simplify

Divide each term in
`{3x ≥ 36 by 3}`

`\green{{(3x)/(3) ≥ (36)/(3)}}`

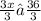
Sixth, Cancel the common factor of 3

Cancel the common factor

`\red{{(3x)/(3)}}` ≥
`\green{{(36)/(3)}}`

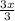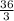
Divide
`{x}` by 1

`\green{{x ≥ (36)/(3)}}`

Lastly, Divide 36 by 3

`\green{\boxed{x ≥ 12}}`

hope it's helps