Question

Determine the points are collinear (1,2) (2,3) and (-2,-11)

Answer 1

Given:

The three points are (1,2), (2,3) and (-2,-11).

To find:

Whether the given points are collinear.

Solution:

Let as consider the three points are A(1,2), B(2,3) and C(-2,-11).

If these three points are collinear, then area of
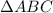`\Delta ABC` must be 0.

Area of
`\Delta ABC`,

`Area=(1)/(2)|x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)|`

`Area=(1)/(2)|1(3-(-11))+2(-11-2)+(-2)(2-3)|`

`Area=(1)/(2)|(3+11)+2(-13)-2(-1)|`

`Area=(1)/(2)|14-26+2|`

`Area=(1)/(2)|-10|`

`Area=(1)/(2)(10)`

`Area=5\\eq 0`

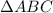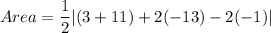
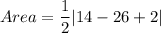
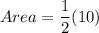
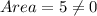
Therefore, the given points are not collinear.