Question

Suppose you invest $3500 at annual interest rate of 2.5 % How much money do you have in the account after five years?

Round two decimal places.

compounded monthly $ ____

compounded weekly $ _____

Answer 1

Please check the explanation.

Explanation:

To find the amount we use the formula:

`A=P\cdot \left(1+(r)/(n)\right)^(nt)`

Here:

A = total amount

P = principal or amount of money deposited,

r = annual interest rate

n = number of times compounded per year

t = time in years

Given

P=$3500

r=2.5%

n=12

t = 5 years

Calculating compounded monthly

After plugging in the values

`A=3500\left(1+(2.5\%\:)/(12)\right)^(12\cdot \:5)`

`A=3500\left(1+(0.025)/(12)\right)^(12\cdot \:5)`

`A=3500\cdot (12.025^(60))/(12^(60))`

`A = 3959.93`

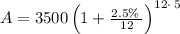
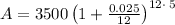
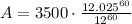
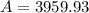
Thus, If you deposit $3500 into an account paying 2.5% annual interest compounded monthly, you will have $3959.93 after five years.

Calculating compounded weekly

n = 52

`A=3500\left(1+(2.5\%\:)/(52)\right)^(52\cdot \:5)`

`A=3500\left(1+(0.025)/(52)\right)^(52\cdot \:5)`

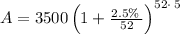
A = 3,965.90

Thus, If you deposit $2000 into an account paying 4.5% annual interest compounded weekly, you will have $3,965.90 after five years.