Answer:
Option A:
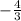 is the correct answer.
is the correct answer.
Explanation:
Given that:
Slope of the line =

Let,
m be the slope of the line perpendicular to the line with slope

We know that,
The product of slopes of two perpendicular lines is equals to -1.
Therefore,
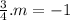
Multiplying both sides by


m =
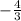
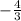 is the slope of the line perpendicular to the line having slope
is the slope of the line perpendicular to the line having slope

Hence,
Option A:
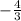 is the correct answer.
is the correct answer.