Question

PLEASE HELP!

A truck pushes a 2030 kg-car with a force 700 N for 5.0 s. The cars starts from rest.
How far will it go?

Answer 1

x = 4.32 [m]

Step-by-step explanation:

We must divide this problem into three parts, in the first part we must use Newton's second law which tells us that the force is equal to the product of mass by acceleration.

∑F = m*a

where:

F = force = 700 [N]

m = mass = 2030 [kg]

a = acceleration [m/s²]

Now replacing:

`F=m*a\\700=2030*a\\a = 0.344[m/s^(2)]`

Then we can determine the final speed using the principle of conservation of momentum and amount of movement.

`(m_(1)*v_(1))+Imp_(1-2)=(m_(1)*v_(2))`

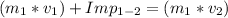
where:

m₁ = mass of the car = 2030 [kg]

v₁ = velocity at the initial moment = 0 (the car starts from rest)

Imp₁₋₂ = The impulse or momentum (force by the time)

v₂ = final velocity after the impulse [m/s]

`(2030*0) + (700*5)=(2030*v_(2))\\3500 = 2030*v_(2)\\v_(2)=1.72[m/s]`

Now using the following equation of kinematics, we can determine the distance traveled.

`v_(2)^(2) =v_(1)^(2)+2*a*x`

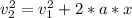
where:

v₂ = final velocity = 1.72 [m/s]

v₁ = initial velocity = 0

a = acceleration = 0.344 [m/s²]

x = distance [m]

`1.72^(2)=0^(2) +(2*0.344*x) \\2.97 = 0.688*x\\x = 4.32 [m]`