Answer:
Average rate of change =

Explanation:
Average rate of change of a function 'f' in the interval a ≤ x ≤ b is given by,
Average rate of change =

We have to find the average rate of change in the interval 20 ≤ x ≤ 65
From the table attached,
f(65) = 32
f(20) = 14
Average rate of change =
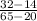
=

=

Therefore, average rate of change in the given interval is
 .
.