The quadratic is continuous over its entire domain, which is to say we can evaluate the limit by direct substitution:
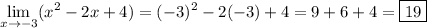
You are mistaking (-3)² for -3². They are not the same number.
(-3)² = (-1 × 3)² = (-1)² × 3² = 1 × 9 = 9
-3² = -1 × 3² = -1 × 9 = -9