Question

Eliminate the parameter “t” for the parametric equations, where 0 < t < 5.

x = √5t

y = 2t — 2


Answers:

A) y = 2/5x^2 — 2
B) y = 5/2X^2 — 5
C) y = 2√5x — 2
D) y = 2/5x^2 — 2

Answer 1

A) y = 2/5x^2 — 2

Explanation:

We are given the parametric equation:

`x=√(5t)`

`y=2t-2`

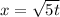
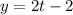
To express y in terms of x, we solve for t in the first equation and substitute in the second equation.

Squaring:

`x^2=(√(5t))^2`

`x^2=5t`

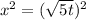
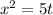
Solving for t:

`\displaystyle t=(x^2)/(5)`

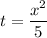
Substituting in the second parametric equation:

`\displaystyle y=2(x^2)/(5)-2`

`\displaystyle y=(2)/(5)x^2-2`

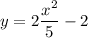
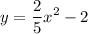
Correct choice: A) y = 2/5x^2 — 2