Question

I NEED HELP PLEASE!!
IM BEGGING YOU!!!

Answer 1

`\displaystyle BC=(27)/(2)`

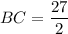
CA = 15.

Explanation:

Similar Triangles

It's given triangle ABC is similar to triangle PQR. Similar triangles have their side lengths proportional by the same scale factor.

To find the scale factor, divide the lengths of two corresponding sides. Sides AB and PQ are corresponding, and the proportion between them is 12/8 = 3/2.

Now we find the required lengths by using the same proportion.

BC is corresponding with QR, thus

`\displaystyle (BC)/(QR)=(3)/(2)`

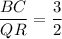
Solving for BC:

`\displaystyle BC=(3)/(2).QR`

`\displaystyle BC=(3)/(2).9=(27)/(2)`

`\mathbf{\displaystyle BC=(27)/(2)}`

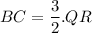
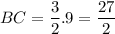
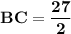
CA is corresponding with RP, thus:

`\displaystyle (CA)/(RP)=(3)/(2)`

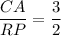
Solving for CA:

`\displaystyle CA=(3)/(2).RP`

`\displaystyle CA=(3)/(2).10`

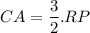
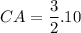
CA = 15