Answer:
A. The coefficients of the sum are whole numbers and the sum is a polynomial.
Explanation:
The approach to demonstrate the closure property for the sum consists in defining what a polynomial is and demonstrate that the sum of two polynomials with integer coefficients is equal to a polynomial with integer coefficients. We proceed to show the proof below:
1)
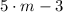 ,
,
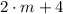 Given
Given
2)
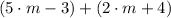 Definition of addition
Definition of addition
3)
![(5\cdot m +2\cdot m ) +[(-3)+4]](https://img.qammunity.org/2021/formulas/mathematics/college/kqsa1l7bfhietxb8lhebgxmqqo0m8u24p5.png) Definition of substraction/Associative and Commutative properties
Definition of substraction/Associative and Commutative properties
4)
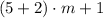 Distributive property/Definition of substraction
Distributive property/Definition of substraction
5)
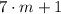 Definition of addition/Result
Definition of addition/Result
Hence, the coefficients of the sum are whole numbers and the sum is a polynomial. The correct answer is A.