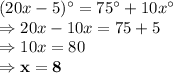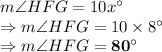Question

If H is in the interior of ∠EFG, m∠EFH = 75°, and m∠HFG = (10x)°, and m∠EFG = (20x − 5)°, then x = ? and m∠HFG = ?

Answer 1

`x=8`

`\m\angle HFG= 80^\circ`

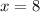
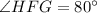
Explanation:

Given that:

Point
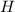`H` is interior of
`\angle EFG`.

`m\angle EFH = 75^\circ\\m\angle HFG = (10x)^\circ\\m\angle EFG = (20x-5)^\circ`

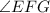To find:

`x = ?\\m\angle HFG = ?`

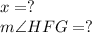
Solution:

First of all, let us represent the given values in the form of a diagram.

Kindly refer to the attached image for the given points and values of angles.

We can clearly see that:

`m\angle EFG = m\angle EFH + m\angle HFG`

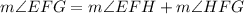
Putting all the values given in above equation, we get:

`(20x-5)^\circ = 75^\circ + 10x^\circ\\\Rightarrow 20x-10x=75+5\\\Rightarrow 10x =80\\\Rightarrow \bold{x =8}`

`m\angle HFG =10x^\circ\\\Rightarrow m\angle HFG =10* 8^\circ\\\Rightarrow m\angle HFG = \bold{80^\circ}`