Question

9.

A constant mass of hydrogen occupies a volume of 4.0 cm at a pressure of 2.0 x 105 Pa
and a temperature of 288K. Calculate the volume of this gas at a pressure of 1.6x 105 Pa
when the temperature is 125K.
(3mks​

Answer 1

Approximately
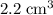`2.2\; \rm cm^(3)` (assuming that this gas is ideal, and that the initial volume of this gas is
`4.0\; \rm cm^(3)`.)

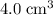Step-by-step explanation:

In this question, both the pressure on the gas and the temperature of the gas have changed. However, the ideal gas laws (Boyle's Law and Charles' Law) requires that only one of the two quantity change at a time. Therefore, consider this change in two steps:

• Change pressure from
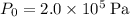  `P_0 = 2.0 * 10^(5)\; \rm Pa` to
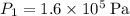  `P_1= 1.6 * 10^(5)\; \rm Pa` while keeping temperature constant at
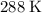  `288\; \rm K`. Find the new volume
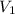  `V_1` using Boyle's Law.

• After that, keep the pressure the same (at
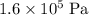  `1.6 * 10^(5)\; \rm Pa`) and change the temperature from
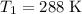  `T_1 = 288\; \rm K` to
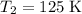  `T_2 = 125\; \rm K`. Find the final volume of the gas
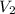  `V_2` using Charles' Law.

Boyle's Law states that for a fixed quantity of some ideal gas, if temperature is held constant, the volume of the gas will be inversely proportional to the pressure on the gas.

Let
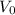`V_0` denote the initial volume of this gas. The question states that at
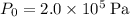`P_0 = 2.0* 10^(5)\; \rm Pa` and
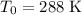`T_0 = 288\; \rm K`, the volume of the gas is
`V_0 = 4.0\; \rm cm^(3)`.

By Boyle's Law, if temperature is held constant (
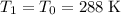`T_1 = T_0 = 288\; \rm K`,) then at
`P_1 = 1.6 * 10^(5)\; \rm Pa`:

`\begin{aligned} V_1 &= V_0 \cdot (P_0)/(P_1) \\ &= 4.0\; \rm cm^3 * (2.0 * 10^(5)\; \rm Pa)/(1.6 * 10^(5)\; \rm Pa) \approx 5.0\; \rm cm^(3) \end{aligned}`.

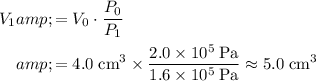On the other hand, Charles' Law suggests that for a fixed quantity of some ideal gas, if the pressure of the gas is held constant, the volume of the gas will be proportional to the temperature (in degree Kelvins) of the gas.

Let
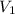`V_1` denote the volume of this gas before the temperature change. At
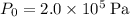`P_0 = 2.0* 10^(5)\; \rm Pa` and
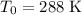`T_0 = 288\; \rm K`, previous calculations show that
`V_0 = 5.0\; \rm cm^(3)`.

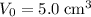By Charles' Law, if the pressure of this gas is held constant (
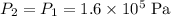`P_2 = P_1 =1.6 * 10^(5)\; \rm Pa`,) then at the new temperature
`T_2 = 125\; \rm K`:

`\begin{aligned} V_2 &= V_1 \cdot (T_1)/(T_0) \\ &= 5.0\; \rm cm^3 * (125\; \rm K)/(288\; \rm K) \approx 2.2\; \rm cm^(3) \end{aligned}`.

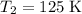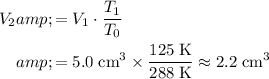Therefore, at
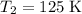`T_2 = 125\; \rm K` and
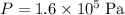`P = 1.6 * 10^(5)\; \rm Pa`, the volume of this gas would be approximately
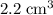`2.2\; \rm cm^(3)`.