Question

At a candy store, Lillian bought 4 pounds of jelly beans and 3 pounds of gummy worms for $34. Meanwhile, Kelsey bought 5 pounds of jelly beans and 3 pounds of gummy worms for $38. How much does the candy cost? A pound of jelly beans costs $insert price, and a pound of gummy worms costs $insert price,

Answer 1

A pound of jelly beans costs $4, and a pound of gummy worms costs $6

Explanation:

Let

Cost of one pound of Jelly beans = x

Cost of one pound of Gummy worms = y

Making equations from the statements:

Lillian bought 4 pounds of jelly beans and 3 pounds of gummy worms for $34:
`4x+3y=34`

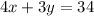
Kelsey bought 5 pounds of jelly beans and 3 pounds of gummy worms for $38:
`5x+3y=38`

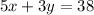
Solving both equations we can found cost of candies.

Let:

`4x+3y=34--eq(1)\\5x+3y=38--eq(2)`

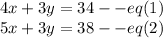
Subtracting both equations to find value of x

`4x+3y=34\\5x+3y=38\\- \ \ \ - \ \ \ \ -\\------\\-x=-4\\x=4`

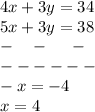
So,we get value of x=4

Now putting value of x in eq(1) to find value of y

`4x+3y=34\\4(4)+3y=34\\16+3y=34\\3y=34-16\\3y=18\\y=18/3\\y=6`

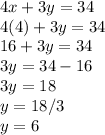
We get value of y=6

Cost of one pound of Jelly beans = x = 4

Cost of one pound of Gummy worms = y = 6

A pound of jelly beans costs $4, and a pound of gummy worms costs $6