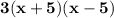Answer:
Factoring the term
 we get
we get
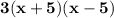
Explanation:
We need to solve the polynomial:

First we see that 3 is common term, so taking 3 as common

We know that 25 = 5x5 = 5²
So, replacing 25 with 5²
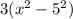
We know that,
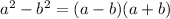
Applying this in our equation:
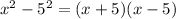
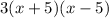
So, factoring the term
 we get
we get