Answer:
f(x) and g(x) are inverse functions
Explanation:
In the two functions f(x) and g(x) if, f(g(x)) = g(f(x)) = x, then
f(x) and g(x) are inverse functions
Let us use this rule to solve the question
∵ f(x) = 3x²
∵ g(x) =
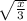
→ Find f(g(x)) by substitute x in f(x) by g(x)
∴ f(g(x)) = 3(
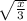 )²
)²
→ Cancel the square root with power 2
∴ f(g(x)) = 3(
 )
)
→ Cancel the 3 up with the 3 down
∴ f(g(x)) = x
→ Find g(f(x)) by substitute x in g(x) by f(x)
∴ g(f(x)) =
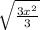
→ Cancel the 3 up with the 3 down
∴ g(f(x)) =

→ Cancel the square root with power 2
∴ g(f(x)) = x
∵ f(g(x)) = g(f(x)) = x
→ By using the rule above
∴ f(x) and g(x) are inverse functions