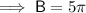Question

What is the period of y = 8 cos (5pie x + 3pie/2)-9
Give an exact value. Units

Answer 1

`\\ \rm\Rrightarrow y=Acos(Bx+C)+D`

• This is the general formula and the period is 2π/B

Now our given function

`\\ \rm\Rrightarrow y=8cos\left(5\pi x+(3\pi)/(2)\right)-9`

On comparing we get

• A=8
• B=5π
• C=3π/2
• D=-9

Period:-

`\\ \rm\Rrightarrow (2\pi)/(B)`

`\\ \rm\Rrightarrow (2\pi)/(5\pi)`

`\\ \rm\Rrightarrow (2)/(5)`

Answer 2

`\textsf{Period}=(2)/(5)`

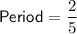
Explanation:

Standard form of a cosine function:

f(x) = A cos(B(x + C)) + D

• A = amplitude (height from the mid-line to the peak)
• 2π/B = period (horizontal distance between consecutive peaks)
• C = phase shift (horizontal shift - positive is to the left)
• D = vertical shift

Given function:

`y= 8 \cos \left(5 \pi x+(3 \pi)/(2)\right)-9`

`\implies y= 8 \cos \left(5 \pi \left(x+(3)/(10)\right)\right)-9`

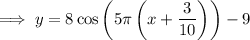
Comparing with the standard form:

`\implies \textsf{B}=5 \pi`

`\implies \textsf{Period}=(2 \pi)/(\sf B)=(2 \pi)/(5 \pi)=(2)/(5)`