Answer:
The value is

Explanation:
From the question we are told that
The sample size is n = 70
The mean is
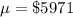
The standard deviation is
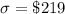
The sample mean is

Generally the number of standard deviations the sample mean is from the mean of the distribution is mathematically represented as
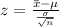
=>
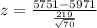
=>
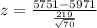
=>
