Answer:
I) The roots are:
First Case:
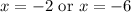
Second Case:
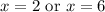
II) Possible values of p are:
First Case:
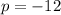
Second Case:
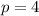
Explanation:
We have the equation:
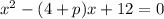
We know that one of the roots is three times the other.
And we want to find the roots of the equation and the possible values for p.
To find our solutions, we can use the process of factoring.
If we have an equation in the form:
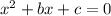
We want to find two numbers, say, k and j, such that:
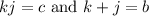
After finding them, we can factor as such:
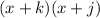
So, -k and -j will be our zeros.
Therefore, for our equation, we will find two numbers k and j such that:

Notice that for the first criterion, we can simply list all the factors of 12.
So, according to the first equation, possible values of k and j are:
1 and 12; 2 and 6; or 3 and 4.
Then, our roots will be -1 and -12; -2 and -6; or -3 and -4, respectively.
However, remember that one of our roots is three times the other.
Therefore, the only candidates that work will be the second option.
Hence, k and j are 2 and 6.
Therefore:
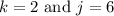
With this information, we can now determine p. Since:

Then it follows that:
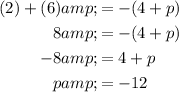
Hence, our equation is:
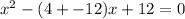
Or, factored:
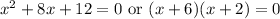
So, our roots are x=-6 and x=-2 when p=-12.
However, we also need to consider the negative factors of 12.
Factors of 12 also include -2 and -6.
Hence, our k and j can also be k=-2 and j=-6.
Then, in this case, our p is :
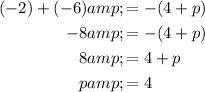
Therefore, for our second case, k=-2 and j=-6. Then p=4. So, our equation is:

Or, factored:
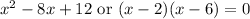
Hence, when p=4, our roots are x=2 and x=6.