Answer:

Explanation:
See attachment for question illustration
From the attachment, we have:

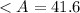
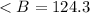
Required
Solve d
First, we need to calculate <C
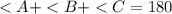 --- angles in a triangle
--- angles in a triangle
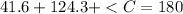
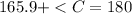
Make <C the subject
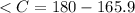
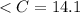
Next, we apply Sine's law to solve for d
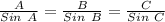
In this case:

Make d the subject
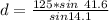

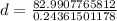


Hence, the base of the lake is approximately 340.7m