Question

What is the area of ABC?

Answer 1

Area of triangle ABC = 10

Option C is correct.

Explanation:

We need to find area of triangle ABC

We are given A(2,1) B(4,7) and C(6,3)

The formula used to find
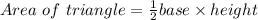`Area \ of \ triangle=(1)/(2)base* height` ABC is:

In the given triangle base= AC and height = BC

First we need to find the distance between A and C and B and C

The formula used to find distance between AC
`Distance=√((x_2-x_1)^2+(y_2-y_1)^2)`

We have
`x_1=2, y_1=1, x_2=6, y_2=3`

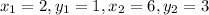
Putting values and finding distance

`Distance=√((x_2-x_1)^2+(y_2-y_1)^2)\\Distance=√((6-2)^2+(3-1)^2)\\Distance=√((4)^2+(2)^2)\\Distance=√(16+4)\\Distance=√(20)`

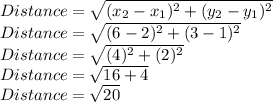
find distance between BC
`Distance=√((x_2-x_1)^2+(y_2-y_1)^2)`

We have
`x_1=4, y_1=7, x_2=6, y_2=3`

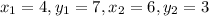
Putting values and finding distance

`Distance=√((x_2-x_1)^2+(y_2-y_1)^2)\\Distance=√((6-4)^2+(3-7)^2)\\Distance=√((2)^2+(4)^2)\\Distance=√(4+16)\\Distance=√(20)`

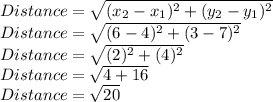
So, We have height =
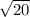`√(20)` and base =
`√(20)`

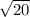
Finding area of triangle

`Area \ of \ triangle=(1)/(2)base* height\\Area \ of \ triangle=(1)/(2)√(20) * √(20) \\Area \ of \ triangle=(1)/(2)* 20\\Area \ of \ triangle=10`

So, Area of triangle ABC = 10

Option C is correct.