Answer:
v_{f}=21.75[m/s] (velocity just before it lands)
t = 2.98 [s]
Step-by-step explanation:
The key to solving this problem is the initial velocity since when the camera falls it is moving up, we will take this velocity as negative and the acceleration gravitation down as positive.
We will use the following equation of kinematics to solve this problem.
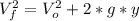
where:
Vf = final velocity [m/s²]
Vo = initial velocity = 7.5 [m/s]
g = gravity acceleration = 9.81 [m/s²]
y = elevation = 27 [m]
![v_(f)^(2) = - (7.5)^(2) +(2*9.81*27)\\ v_(f)^(2) = 473.5\\v_(f)=√(473.5)\\v_(f)=21.75[m/s]](https://img.qammunity.org/2021/formulas/physics/high-school/7ff0sq93gohqtzyetrd7p1pwiprpt8twua.png)
Now using the following equation of kinematics, we can calculate the time that the fall lasts.
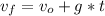
![21.75 = -7.5 +9.81*t\\9.81*t = 29.25\\t = 2.98[s]](https://img.qammunity.org/2021/formulas/physics/high-school/rtsgk2slp4gtongacjszjnc2yfgcn6hpjq.png)