Answer:
The perimeter of triangle XYZ is 54 cm
Explanation:
- In similar triangles, their corresponding sides are proportional, which means the ratios of the corresponding sides are equal
- Their perimeters have the same ratio as their corresponding sides, which means P1 = r × P2, where r is the ratio of similarity
- Their areas have the square of the ratio as their corresponding sides, which means A1 = r² × A2
∵ Δ ABC is similar to Δ XYZ
∵ The longest side in Δ XYZ is 25.2 cm
→ That means its corresponding side in Δ ABC is the longest side
∵ AB = 18 cm, BC = 30 cm, CA = 42 cm
∴ The longest side in Δ ABC is CA
∴ CA is the corresponding side of the side of length 25.2 cm
∴ The ratio of similarity =
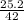 = 0.6 ⇒ (r)
= 0.6 ⇒ (r)
∵ The perimeter of Δ ABC = AB + BC + CA
∴ The perimeter of Δ ABC = 18 + 30 + 42
∴ The perimeter of Δ ABC = 90 cm
→ Use the 2nd fact above
∵ P of Δ XYZ = r × P of Δ ABC
∵ r = 0.6 and P of Δ ABC = 90
∴ The perimeter of Δ XYZ = 0.6 × 90
∴ The perimeter of Δ XYZ = 54 cm