Question

At what point does the graph of 3x + 4y = 15 intersect the graph of x^2 + y^2 = 9? Express any non-integer coordinate as a common fraction.

Answer 1

12 and 36 and 13

Answer 2

If you need an explanation;

let's first isolate x in 3x + 4y = 15 ---

x = (15 - 4y) / 3

now substitute as x in the second equation provided ---

((15 - 4y) / 3)^2) + y^2 = 9,

((15 - 4y) / 3)^2) +( 3y/3)^2 = 9,

((15 - 4y)^2 + (3y/3)^2)/3^2 = 9,

225 - 120y +25y^2/9 = 9,

225 - 120y +25y^2 = 81,

25y^2 - 120y + 144 = 0

we now solve using the quadratic formula ---

`y_(1,\:2)=(-\left(-120\right)\pm √(\left(-120\right)^2-4\cdot \:25\cdot \:144))/(2\cdot \:25)\\=(-\left(-120\right)\pm √(0))/(2\cdot \:25)\\=(-\left(-120\right)\pm √(0))/(2\cdot \:25)\\\\= 12/5`

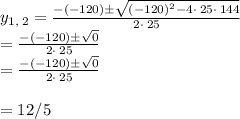
So then x = (15 - 4*12/5)/3 = 9/5. Our solution = (9/5, 12/5)