Question

A transportation worker takes a simple random sample of 105 toll booth receipts for one day and finds that the mean is $9.45 with a standard deviation of $2.41. what is the 99% confidence interval for the mean amount of all the receipts that day?

Answer 1

The 99% confidence interval for the mean amount of all toll booth receipts is calculated using the formula for a large sample size and the z-score for a 99% confidence level. The resulting interval is ($8.84, $10.06).

Step-by-step explanation:

The question asks us to calculate the 99% confidence interval for the mean amount of all toll booth receipts based on a simple random sample. To compute the confidence interval for a mean, we use the formula:
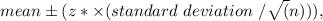`mean \± (z* * (standard \ deviation \ / \sqrt(n))),` where z* is the z-score corresponding to the desired confidence level, standard deviation is the sample standard deviation, and n is the sample size. Since the sample size is over 30, we'll use the z-distribution which is appropriate for large samples according to the Central Limit Theorem.

For a 99% confidence interval, the z-score is approximately 2.576. Thus, the confidence interval is calculated as
`\$9.45 \± (2.576 * ($2.41 / \sqrt(105))).`

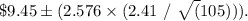
The computation yields the confidence interval as: $9.45 ± 0.61, or ($8.84, $10.06). We are 99% confident that the true mean of all receipts is between $8.84 and $10.06.

Answer 2

esa preguntas en inglés yo hablo español burro