Answer:
Z-score = 0.9450
Explanation:
Step(i):-
mean height of pine tree (x₁⁻) = 52
standard deviation of pine tree σ₁ = 3
Mean height of Jupiter tree ((x₂⁻) = 22
standard deviation of Jupiter tree σ₂ = 5
Size of first sample n₁ = 48
size of second sample n₂ = 33
Step(ii):-
Z-score

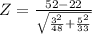
Z = 0.9450