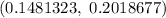Question

To estimate a proportion of students with a grade point average of 3.7 and higher in a college, a random sample of 200 students was taken and the number of successes counted to be 35. The 95% confidence interval for a population proportion is:

Answer 1

Answer:
`(0.1481323,\ 0.2018677)`

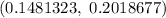
Explanation:

The confidence interval for population proportion :

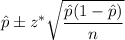`\hat{p}\pm z^*\sqrt{\frac{\hat{p}(1-\hat{p})}{n}}` , where n= sample size,
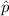`\hat{p}` = sample proportion , z*= Critical z-value.

Let p = population proportion of successes.

Given: n= 200 ,
`\hat{p}=(35)/(200)=0.175`

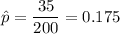
Critical z value for 95% confidence = 1.96

The 95% confidence interval for a population proportion is:

`0.175\pm (1.96)\sqrt{((0.175)(1-0.175))/(200)}\\\\=\ 0.175\pm (1.96)√(0.000721875)\\\\= 0.175\pm (1.96)(0.0268677)\\\\=(0.175-0.0268677,0.175+0.0268677)\\\\= (0.1481323,\ 0.2018677)`

Required confidence interval:
`(0.1481323,\ 0.2018677)`