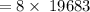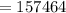Answer:
Please check the explanation!
Explanation:
Given the expression what Lizzet said was correct
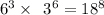
No, she is not right. Let me solve the expression
Let us solve the expression
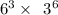
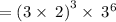

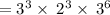 ∵
∵
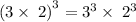
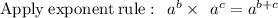
 ∵
∵

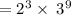
Therefore, the correct expression will be:

which can be further simplified such as: