Question

Find the equation of the line that passes through the points (-2, 6) and (2-6). Write your answer in slope

intercept form. (Y=mx+b)

What is the y=mx+b?

Answer 1

y = -3x

Explanation:

Slope-intercept form:

Slope formula:
`(y2-y1)/(x2-x1)`

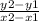
Given points: (-2, 6), (2, -6)

(2, -6) = (x1, y1)

(-2, 6) = (x2, y2)

To write the equation in y = mx + b form, we need to know the slope(m) and the y-intercept(b). To find the slope, input the two given points into the slope formula:

`(6-(-6))/(-2-2)`

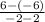
Simplify:

6 - (-6) = 6 + 6 = 12

-2 - 2 = -4

`(12)/(-4) = -(3)/(1) =-3`

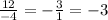
The slope of the equation is -3. To find b, we can input the value of the slope and one point (in this example I'll use (2, -6) into the equation:

-6 = -3(2) + b

-6 = -6 + b

0 = b

The y-intercept is 0. Now that we know the slope and y-intercept, we can write the equation:

y = mx + b

y = -3x + 0

y = -3x

The equation written in slope-intercept form is y = -3x.