Answer:
Its better to invest at 2.45% interest rate compounded daily.Step-by-step explanation:
We are given
Principal Amount = $350,000
Time t = 3 years
We need to determine which investment will yield greater return
a) 2.5% compounded monthly
b) 2.45% compounded daily
The formula used will be:
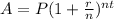
a) 2.5% compounded monthly
We have P=$350,000 t= 3 years, r = 2.5 or 0.025 and n= 12
Putting values and finding A
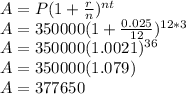
So, the return of investment in 3 years would be $377650 if interest rate is 2.5% compounded monthly.
a) 2.45% compounded daily
We have P=$350,000 t= 3 years, r = 2.5 or 0.025 and n= 365
Putting values and finding A
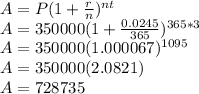
So, the return of investment in 3 years would be $728735 if interest rate is 2.45% compounded daily.
So, if we invest 2.45% interest rate that is compounded daily we will make $728735 while if we invest 2.5% interest rate that is compounded monthly we will make $377650
So, its better to invest at 2.45% interest rate compounded daily.