Question

Which value or values for the variable x from the set below will make 4.5 + 1.2x ≥ 10 - x true? {3, 4, 5, 6}

1. only 3
2. 3 and 4
3. 3,4, and 5
4. all values in the set

Answer 1

Option 4 is correct option.

Explanation:

We need to find the value for the variable x from the set {3,4,5,6} below will make
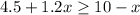`4.5 + 1.2x \geq 10 - x` true

First we solve the inequality to find values for x

`4.5 + 1.2x \geq 10 - x`

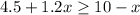
Adding x on both sides

`4.5 + 1.2x+x \geq 10 - x+x\\4.5+2.5x\geq 10`

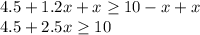
Subtracting 4.5 from both sides

`4.5+2.5x-4.5\geq 10-4.5\\2.5x\geq 5.5\\`

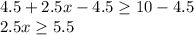
Divide both sides by 2.5

`(2.5x)/(2.5) \geq (5.5)/(2.5)\\x\geq 2.2`

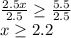
So, after solving inequality we get x ≥ 2.2

So, all values greater than 2.2 can be considered true. In the set given {3,4,5,6} all values are greater than 2.2 so all values in the set are considered true for
`4.5 + 1.2x \geq 10 - x`

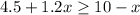
Option 4 is correct option.