Question

A corner store sells two kinds of baked goods: cakes and pies. A cake costs $5 and a pie costs $4. In one day, the store sold 10 baked goods for a total of $46.

a. Write a system of equations to represent this situation.

b. Show work, using substitution, to determine how many cakes were sold.

Answer 1

a. The system of equations is:

c+p=10

5c+4p=46

b. 6 cakes were sold.

Explanation:

Let p be the number of pies and c be the number of cakes then according to given statements

`c+p=10\ \ \ eqn\ 1\\5c+4p=46\ \ \ eqn\ 2`

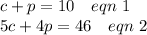
Using eqn 1

c= 10-p

Putting in eqn 2

`5(10-p)+4p=46\\50-5p+4p=46\\50-p = 46\\-p=46-50\\-p=-4\\p=4`

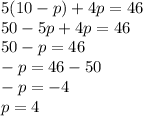
Putting p=4 in eqn 1

`c+p=10\\c+4=10\\c=10-4\\c=6`

Hence,,

a. The system of equations is:

c+p=10

5c+4p=46

b. 6 cakes were sold.