Question

Perpendicular to y=2x+9 and has the point (4,-1)

Answer 1

`\sf y=-(1)/(2)x+1`

Explanation:

Slope-intercept form: y = mx + b

where:

• m is the slope
• b is the y-intercept (when x = 0)

Given: y = 2x + 9

where:

• 2 is the slope
• (0, 9) is the y-intercept

Note:

Perpendicular lines have slopes that are negative reciprocals of each other.

`\sf \textsf{a slope of 2} \implies \textsf{would have a negative reciprocal of} -(1)/(2)`

`\textsf{Perpendicular line:}\ \sf y=-(1)/(2)x+b`

Substitute the given point into the the equation to find the value of b:

`\sf \sf y=-(1)/(2)x+1\\\\-1=-(1)/(2)(4)+b\\\\-1=-2+b\\\\-1+2=-2+2+b\\\\1=b`

`\textsf{Perpendicular line:}\ \sf y=-(1)/(2)x+1`

• slope of ½
• y-intercept of (0, 1)

Answer 2

Answer: y =
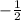`-(1)/(2)`x + 1

Explanation:

First, we will find the slope. The slope of perpendicular lines are negative reciprocals.

In this case, the first slope is 2. The negative of 2 is -2, and the reciprocal of -2 is
`-(1)/(2)`.

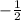Now, we will plug in this new slope, the point given, and solve for the b, or the y-intercept.

y = mx + b

(-1) = (
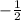`-(1)/(2)`)(4) + b

-1 = -2 + b

1 = b

Lastly, we will write our equation.

y = mx + b

y =
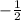`-(1)/(2)`x + 1

The line is y =
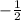`-(1)/(2)`x + 1, or y = 1 -
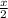`(x)/(2)`.