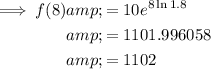Question

NO LINKS!! Given the exponential relationships below, find the following​

Answer 1

Exponential Function

General form of an exponential function with base
`e`:

`f(x)=Ae^(kx)`

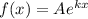
where:

• A is the y-intercept
• e (Euler's number) is the base
• k is some constant

Question 30

The curve crosses the y-axis at y = 40. Therefore, A = 40.

Substitute the found value of A into the formula along with (1, 56) and solve for
`k`:

`\begin{aligned}f(x) & =Ae^(kx)\\\implies 56 & =(40)e^(k)\\e^k & =(56)/(40)\\k & =\ln (1.4)\end{aligned}`

`\textsf{Equation}: \quad f(x)=40e^(x\ln 1.4)`

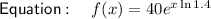
To find the population in 10 years, substitute
`x = 10` into the found equation:

`\begin{aligned}\implies f(10)&=40e^(10\ln 1.4)\\ & =1157.01862\\ & =1157\end{aligned}`

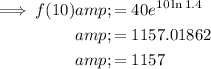
Question 31

The curve crosses the y-axis at y = 10. Therefore, A = 10.

Substitute the found value of A into the formula along with (1, 18) and solve for
`k`:

`\begin{aligned}f(x) & =Ae^(kx)\\\implies 18 & =(10)e^(k)\\e^k & =(18)/(10)\\k & =\ln (1.8)\end{aligned}`

`\textsf{Equation}: \quad f(x)=10e^(x\ln 1.8)`

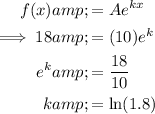
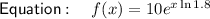
To find the population in 8 years, substitute
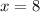`x = 8` into the found equation:

`\begin{aligned}\implies f(8)&=10e^(8\ln 1.8)\\ & =1101.996058\\ & =1102\end{aligned}`