Question

A triangle has sides with lengths of 4 inches, 8 inches, and 9 inches. Is it a right triangle?

Answer 1

No, its not a right triangle

Explanation:

`4^(2) +8^(2) =9^(2)`

16 + 64 = 81

80 = 81

Answer 2

`\boxed {\boxed {\sf No, \ not \ a \ right \ triangle}}`

Explanation:

If a triangle is a right triangle, it will satisfy Pythagorean's Theorem.

`a^2+b^2=c^2`

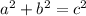
When a and b are the legs of the triangle and c is the hypotenuse.

4 and 8 would be the legs of the triangle. 9 would be the hypotenuse because it is the longest side.

`(4)^2 + (8)^2 = (9)^2`

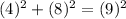
Solve the exponents.

`16+64=81`

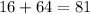
Add on the left side of the equation.

`80=81\\80 \\eq 81`

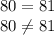
80 is not equal to 81. The triangle does not satisfy Pythagorean's Theorem, so it cannot be a right triangle.