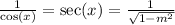Answer:
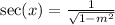
Explanation:
We know that:
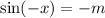
First, since sine is an odd function, we can move the negative outside:
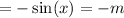
Divide both sides by -1:
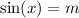
We will now use the Pythagorean Identity:

Substitute m for sine:
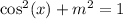
Solve for cosine:
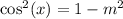
Take the square root of both sides:

Since x is an acute angle, cosine will always be positive. Thus:
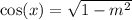
Take the reciprocal of both sides. Hence: