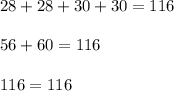Question

Perimeter =116
Yes plz need help

Answer 1

`x=30`

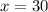
Explanation:

First, let us notice that the ticks on the side of this quadrilateral indicate that the lengths of the sides are equal.

From this, we can create the following equation based on the perimeter of this shape.

`28+28+x+x=116`

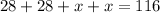
Now, we can solve this equation for x by first combining like terms

`2x+56=116`

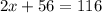
Now, we can subtract 56 from each side

`2x=60`

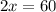
And now divide each side by 2

`x=30`

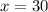
Just to check out answer, we can plug this x-value into our original perimeter equation to see if it is correct

`28+28+30+30=116\\\\56+60=116\\\\116=116`