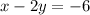Question

100 points, pls help asap

Write the standard form of the line that contains a slope of
1/2 and y- intercept of 3. include your work in your final answer.

Answer 1

`x-2y=-6`

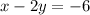
Explanation:

The line contains a slope of 1/2 and has a y-intercept of 3.

And we want to write this in standard form.

Let’s first write it in slope-intercept form:

`y=mx+b`

Where m is the slope and b is the y-intercept.

So, substitute 1/2 for m and 3 for b. This yields:

`y=(1)/(2)x+3`

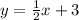
We will convert this to standard form. The standard form is:

`Ax+By=C`

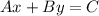
Where A, B, and C are all integers, and A is positive.

We have the slope-intercept equation:

`y=(1)/(2)x+3`

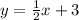
So, let’s multiply our slope-intercept equation by 2 to remove the fraction. This gives us:

`2y=x+6`

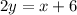
Now, subtract x from both sides:

`-x+2y=6`

The coefficient of x should be positive. So, multiply both sides by -1:

`x-2y=-6`

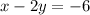
Hence, our standard form is:

`x-2y=-6`