Question

If f(x)=8x+2, find the inverse *

Answer 1

♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️

To find the inverse first we need to solve the equation for x ,

Then put x instead of y .

_________________________________

Let's do it...

`f(x) = 8x + 2`

`y = 8x + 2`

`8x + 2 = y`

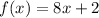
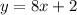
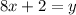
Subtract sides 2

`8x + 2 - 2 = y - 2`

`8x = y - 2`

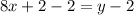
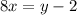
Divide sides by 8

`(8x)/(8) = (y - 2)/(8) \\`

`x = (y - 2)/(8) \\`

♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️

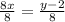
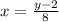
Thus ;

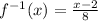
`{f}^( - 1) (x) = (x - 2)/(8) \\`

♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️