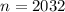Question

In a random sample of 500 college students, 23% say that they read or watch the news every day. Develop a 90% confidence interval for the proportion of all students who read or watch the news on a daily basis. Interpret your results. If you wanted to develop a 95% confidence interval with a margin of error of .01, how many students would need to be surveyed?

Answer 1

The 90% confidence interval is
`0.199 <  p < 0.261`

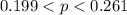
The sample size to develop a 95% confidence interval is
`n = 2032`

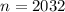
Explanation:

From the question we are told that

The sample size is n =500

The sample proportion is
`\^ p = 0.23`

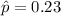
From the question we are told the confidence level is 90% , hence the level of significance is

`\alpha = (100 - 90 ) \%`

=>
`\alpha = 0.10`

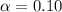
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  1.645`

Generally the margin of error is mathematically represented as

`E =  Z_{(\alpha )/(2) } * \sqrt{(\^ p (1- \^ p))/(n) }`

=>
`E =  1.645 * \sqrt{(0.23 (1- 0.23))/(500) }`

=>
`E =  0.03096`

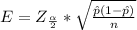
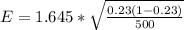
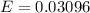
Generally 90% confidence interval is mathematically represented as

`\^ p -E <  p <  \^ p +E`

=>
`0.23  -0.03096  <  p < 0.23  +  0.03096`

=>
`0.199 <  p < 0.261`

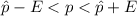
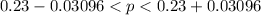
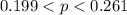
From the question we are told the confidence level is 95% , hence the level of significance is

`\alpha = (100 - 95 ) \%`

=>
`\alpha = 0.05`

Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  1.96`

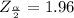
The margin of error is given as
`E = 0.01`

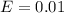
Generally the sample size is mathematically represented as

`n = [\frac{Z_{(\alpha )/(2) }}{E} ]^2 * \^ p (1 - \^ p )`

=>
`n = [(1.96 )/(0.01) ]^2 *0.23 (1 - 0.23 )`

=>
`n = 2032`