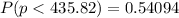Question

Suppose 52R% of the population has a college degree. If a random sample of size 808808 is selected, what is the probability that the proportion of persons with a college degree will be less than 54T%

Answer 1

The probability is
`P( p < 435.82 ) = 0.54094`

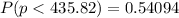
Explanation:

From the question we are told that

The population proportion is p = 54% = 0.54

The sample size is n = 808

Generally the distribution of the population with college degree follows a binomial distribution

i.e

`X  \~ \ \ \  B(n , p)`

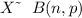
Generally the mean is mathematically represented as

`\mu = n * p`

=>
`\mu = 808 * 0.52`

=>
`\mu = 420.16`

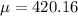
Generally the standard deviation is mathematically represented as

`\sigma = √(np(1- p ))`

=>
`\sigma = √(808 * 0.52(1- 0.52 ))`

=>
`\sigma = 14.2`

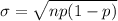
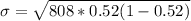
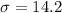
Generally 54% of the population proportion is

`\^ p = 0.54 * 808`

=>
`\^ p = 436.32`

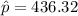
Generally by normal approximation of the binomial distribution the probability that the proportion of persons with a college degree will be less than 54% is mathematically evaluated as

`P(p < \^ p ) = P((p - \mu )/(\sigma ) < (\^ p - \mu )/(\sigma ) )`

`(X -\mu)/(\sigma )  =  Z (The  \ standardized \  value\  of  \ X )`

=>
`P(p < 436.32 ) = P( Z < (436.32 - 420.16 )/(14.20 ) )`

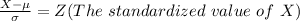
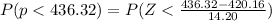
applying continuity correction

`P(p < (436.32-0.5) ) = P( Z < ((436.32-0.5) - 420.16 )/(14.20 ) )`

=>
`P(p < (435.82 ) = P( Z < (435.82 - 420.16 )/(14.20 ) )`

=>
`P(p < (435.82 ) = P( Z < 0.1028 )`

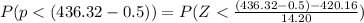
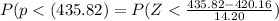
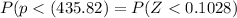
From the z table the area under the normal curve to the left corresponding to 0.1028 is

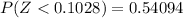
`P( Z < 0.1028 ) = 0.54094`

=>
`P( p < 435.82 ) = 0.54094`