Question

An inductor is connected to a 26.5 Hz power supply that produces a 41.2 V rms voltage. What minimum inductance is needed to keep the maximum current in the circuit below 126 mA?

Answer 1

The minimum inductance needed is 2.78 H

Step-by-step explanation:

Given;

frequency of the AC, f = 26.5 Hz

the root mean square voltage in the circuit,
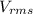`V_(rms)` = 41.2 V

the maximum current in the circuit, I₀ = 126 mA

The root mean square current is given by;

`I_(rms) = (I_o)/(√(2) ) \\\\I_(rms) = (126*10^(-3))/(√(2) )\\\\I_(rms) =0.0891 \ A`

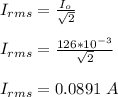
The inductive reactance is given by;

`X_l = (V_(rms))/(I_(rms)) \\\\X_l= (41.2)/(0.0891)\\\\X_l = 462.4 \ ohms`

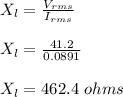
The minimum inductance needed is given by;

`X_l = \omega L\\\\X_l = 2\pi fL\\\\L = (X_l)/(2\pi f)\\\\L = (462.4)/(2\pi *26.5)\\\\L = 2.78 \ H`

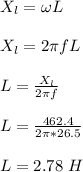
Therefore, the minimum inductance needed is 2.78 H