Question

Zinc has a work function of 4.3 eV. a. What is the longest wavelength of light that will release an electron from a zinc surface? b. A 4.7 eV photon strikes the surface and an electron is emitted. What is the maximum possible speed of the electron?

Answer 1

a

`\lambda_(long) = 288.5 \ nm`

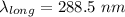
b

The velocity is
`v = 3.7 *0^(5) \ m/s`

Step-by-step explanation:

From the question we are told that

The work function of Zinc is
`W = 4.3 eV`

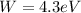
Generally the work function can be mathematically represented as

`E_o = (hc)/(\lambda_(long))`

=>
`\lambda_(long) = (hc)/(E_o)`

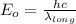
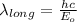
Here h is the Planck constant with the value
`h = 4.1357 * 10^(-15) eV s`

and c is the speed of light with value
`c = 3.0 *10^(8) \ m/s`

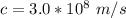
So

`\lambda_(long) = (4.1357 * 10^(-15) * 3.0 *10^(8))/(4.3)`

=>
`\lambda_(long) = 2.885 *10^(-7) \ m`

=>
`\lambda_(long) = 288.5 \ nm`

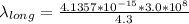
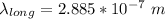
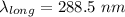
Generally the kinetic energy of the emitted electron is mathematically represented as

`K = E -E_o`

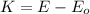
Here E is the energy of the photon that strikes the surface

So

`E- E_o = (1)/(2) m * v^2`

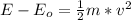
Here m is the mass of electron with value
`m = 9.11*10^(-31 ) \ kg`

Generally
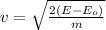
`1 ev = 1.60 *10^(-19) \ J`

=>
`v = \sqrt{ (2 (E - E_o ) )/( m ) }`

=>
`v = \sqrt{ (2 (4.7 - 4.3 )* 1.60 *10^(-19) )/( 9.11 *10^(-31) ) }`

=>
`v = 3.7 *0^(5) \ m/s`