Question

Any ideas for this question

Answer 1

Answer: 36pi (choice b)

===========================================================

Step-by-step explanation:

Let x = length of side BC

This is the height of the cylinder. Think of a can that is laying on its side. The radius of this can or cylinder is CD

If point B has x coordinate of 12, and BC is 12 units long, this must mean point C has x coordinate of 12-x. This is plugged into the function to show that CD has a length of exactly
`√(12-x)`

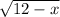
This is the radius of the cylinder

The volume of a cylinder is
`V = \pi*r^2*h`

Plug in the radius and height mentioned to get this function in terms of x

`V = \pi*\left(√(12-x) \ \right)^2*x`

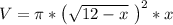
That simplifies to

`V = \pi(12-x)x`

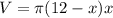
or

`V = \pi(12x-x^2)`

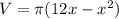
Ignore the pi portion for now.

We wish to maximize the function f(x) = 12x-x^2

Use either calculus (specifically derivatives) or a graphing calculator to find that the vertex is at (6, 36)

This means x = 6 leads to the largest f(x) value being 36.

Therefore, the volume V is maxed out when x = 6 and we get a max volume of 36pi cubic units.