Question

Compare the equations represented in the table, equation, and graph over

the interval
[-5, 3]. Which function is increasing the fastest?

Answer 1

Tabled Function

Explanation:

To determine which function is increasing the fastest over the interval [-5, 3], we need to calculate and compare each function's average rate of change over the given interval.

The average rate of change of function f(x) over the interval a ≤ x ≤ b is given by:

`(f(b)-f(a))/(b-a)`

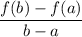
Given interval: -5 ≤ x ≤ 3

Therefore, a = -5 and b = 3

Tabled function

`f(3)=7`

`f(-5)=-17`

`\implies \textsf{Average rate of change}=(f(3)-f(-5))/(3-(-5))=(7-(-17))/(3+5)=3`

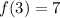
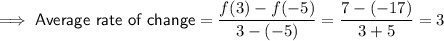
Equation: y = x² - 2

`f(3)=(3)^2-2=7`

`f(-5)=(-5)^2-2=23`

`\implies \textsf{Average rate of change}=(f(3)-f(-5))/(3-(-5))=(7-23)/(3-(-5))=-2`

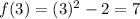
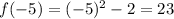
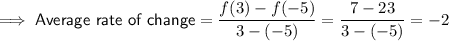
Graphed function

From inspection of the graph:

`f(3)\approx8`

`f(-5) \approx 0`

`\implies \textsf{Average rate of change}=(f(3)-f(-5))/(3-(-5)) \approx (8-0)/(3-(-5))=1`

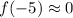
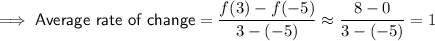
Therefore, the Tabled Function has the greatest average rate of change in the interval [-5, 3] and so it is increasing the fastest.