Answer:
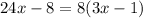
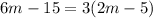
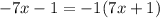
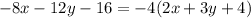
Explanation:
Required
Select which of the equation is true
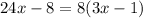
Open the bracket on the right hand side


Both sides of the equation are equal.
Hence, this equation is true
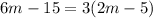
Open the bracket on the right hand side
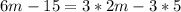
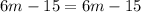
Both sides of the equation are equal.
Hence, this equation is true
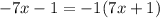
Open the bracket on the right hand side
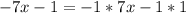
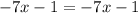
Both sides of the equation are equal.
Hence, this equation is true
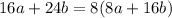
Open the bracket on the right hand side
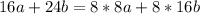
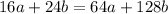
Both sides of the equation are not equal.
Hence, this equation is false
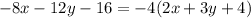
Open the bracket on the right hand side
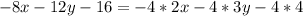
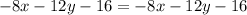
Both sides of the equation are equal.
Hence, this equation is true